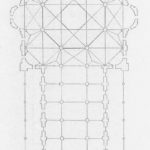
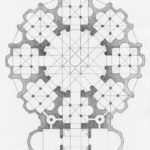
The Octagon in Leonardo’s Drawings
Abstract. Mark Reynolds presents a study on Leonardo’s abundant use of the octagon in his drawings and architectural renderings. Specifically, he focuses on Leonardo’s applications of the octagon: in his studies and sketches of the centralized church, and for which we can find influences specifically from Brunelleschi, as well as from other fifteenth-century architects working with this type of religious structure; in his almost obsessive and frequently repetitious drawing of octagonal shapes and forms in his notebooks throughout his career; in his project for a pavilion while with the Sforzas in the last part of his period in Milan. Also examined are ways to develop the modules to accommodate √2 and the theta rectangles. The application of the modular units, so far, have been within the square and its gridwork, but as the octagon has traditionally been used in the development of both the circle and the square this shape is an interesting challenge in terms of linking the two dimensional surface to the three dimensional forms we are planning to generate. The object is to provide us with more insight as to why the octagon held so much fascination for Leonardo as one of the ultimate geometric expressions of grandeur and practicality in spatial organization, design, and development.
Often in Leonardo’s drawings of octagons, precise geometric constructions were lacking, the master’s approach: freehand. The author seeks to find if Leonardo’s sketches can be put to the rigors of strict geometric construction, and still be viable as accurate renderings of octagonal geometric spaces with his own geometric constructions of those same spaces.
Geometry existed before the creation of things, as eternal as the Spirit of God; it is God Himself and He gave Him the prototypes for the creation of the world. – Johannes Kepler – Harmonices Mundi, 1619
Although God Himself delights in the odd number of the Trinity, nonetheless He unfolds Himself profoundly through the quadrinity in all things.” – Giordano Bruno – On the Monas, ca.1598 – Hamburg Edition, 1991
I Introduction
It is obvious to anyone spending time looking through Leonardo’s sketchbooks that he drew octagons and octagonal systems almost obsessively throughout his long artistic career (fig. 1).1 Even when he did not draw the completed octagon, we still find Leonardo’s preoccupation with octagonal geometry. In rapid sketches and in considered drawings, from floor plans to mechanical devices, we find octagonal shapes taking precedence over other geometric systems. At other times, Leonardo’s interest in the square and its diagonal, requisites for √2 geometry and the octagon, can also be found scattered throughout his studies. One well-known example is in the Codex Atlanticus (Cod. Atl), ff. 190 v-b. It shows the head of a warrior for The Battle of Anghiari, with notes on geometry that include several square and diagonal constructions. There is a cube with a diagonal on one of the square faces. The cube also has an internal diagonal that runs inside the form, from front corner to rear corner of the cube, which demonstrates the √2 and √3 lengths within the form.
Fig. 1. Octagon and octagonal star, Cod. Atl. fol. 223 r-a, after Leonardo; pen and ink and conte crayon on ochre paper. All figures are by the author, after Leonardo.
(The construction demonstrates that Leonardo’s explorations were far more that rudimentary.) A drawing for the plan of the city of Imola, in 1502 (Windsor, RL 12284) shows a plan view of the city drawn in a circle divided into eight parts (with four subdivisions of each of the eight sections). Several drawings of octagon-based fortifications done in 1504 can be found in Cod. Atl. folio 48, v-a. Cod. Atl. f 286 r-a, of technological studies and wooden architecture (an “anatomy theatre”?), shows a circle divided into eight parts, each containing its own circle. There is also a famous sheet of sketches for the Last Supper and geometrical drawings in the Royal Library (Windsor RL, 12542).
Fig. 2. Construction for an octagon inscribed in a circle, Windsor 12542, .after Leonardo; silverpoint
Predominating the middle of the page is a circle, drawn with compasses. At its base, we find the construction for generating an octagon (fig. 2) that would fill this circle, but Leonardo was satisfied not to complete the polygon within that circle. Perhaps he saw it completed in his own mind and found no need to draw it out, or perhaps this was his way of showing a most essential part of the octagon: how it can be generated within the circle from the diagonal of a generating square. It is striking to see the rapid studies for Christ and His Apostles juxtaposed with this exacting geometric construction. There are also other, smaller octagons on the page. This list of octagons could go on for quite some time, but for us, the question is why Leonardo seemed to favor this shape over other geometric shapes, and why he investigated octagonal systems as the basis for centrally planned architecture above all others.
Da Vinci used the octagon in his architectural drawings almost to the exclusion of any other, save a rare foray into a hexagon or an occasional dodecagon.[1] There were barely any architectural investigations done with the pentagonal system, other than a couple of fortress and defense constructions. There are times when Leonardo explores hexagonal geometry, occasionally in a temple plan, but more often when working with tessellations and patterns, including knot designs and embroidery. We do also find drawings relating to the circle and the square. Yet the octagon is related to these two shapes, and so, we are left with the predominance of the octagon and Leonardo’s relentless curiosity about it. But why choose the octagon over the other regular polygons when he knew of them all, how to draw them, their armatures and proportioning systems? Perhaps we can find a few possible answers from looking at the time in which he lived, and the prevailing state of the world of art, architecture, and geometry into which he was introduced and that he developed.
II. On the Nature of the Octagon
In art schools, architecture and design students are required to have two right angled triangles, traditionally known as “set squares.” They are: 1.) the 45°/45°/90° triangle, and, 2.) the 30°/60°/90° triangle. For centuries, these tools have been traditionally used for ad Quadratum (“from the Square,” 45/45/90) and ad Triangulum (“from the Triangle,” 30/60/90) geometry. The two systems of proportioning were learned early in one’s training in geometric construction, and remained part of the geometer’s toolbox throughout one’s career. Although a somewhat broad generalization, we may say that we usually find the development of ad Triangulum in Eastern cultures, Byzantium, and in the early part of the European Middle Ages, while ad Quadratum seems to have found more of a home in Ancient Greece and Rome[2]. The square, ‘square root two’ geometry, and the theta (√2 + 1) rectangle continued to be developed and utilized in European countries well into the Renaissance. (The subcontinent and China had always had an interest in the application of ad Quadratum geometry as well.) The square, both as a system in and of itself, and as an integral part of all rectangles, was commonplace, and frequently, the circle and the square could be found in various combinations with each other and employed in various rectangular ratios. But it was Leonardo’s ability to combine these other shapes with the octagon, and how these earlier uses of octagonal systems and symmetries may have influenced his own work that is of particular note. It is with this backdrop of ad quadratum geometry in Europe then that we begin our discussion of the octagon.
In the quattrocento, geometry, symbolism, and the spiritual were still closely bound; studies on this subject are sometimes referred to as philosophical, sacred, or contemplative geometry. In this field of inquiry, the octagon was seen symbolically as the “intermediary”- the connecting shape – between the circle and the square[3]. The three shapes were traditionally drawn vertically, with the circle at the top representing the cosmos, and the square placed at the bottom to represent the earth. The octagon was drawn in between as that shape that connects the two. Occasionally, the octagon was viewed as a symbol for infinity. It was suggested that the octagon is a circle attempting to become a square, and a square attempting to become a circle. This concept was fully realized in the development of the Hindu vasta-purusha mandala, an ancient type of architecture.[4] Mandala constructions combining the circle and the square usually result in the predominance of four or eight side figures. In China, the octagon represented a complex series of references, but we can say that its meaning was chiefly that of warding off evil entities and being a vehicle for the advent of good health and good fortune. In the number symbolism of Medieval Europe, eight was seen as representing cosmic balance and eternal life.[5] Related to this, the octagon also had deep significance for the Roman Catholic Church. The octagon and the star octagram were religious symbols for rebirth and resurrection. It was used in Baptismal Fonts in many churches, large and small, throughout the entire country. J.C. Cooper, in, An Illustrated Encyclopedia of Traditional Symbols, states that Baptismal fonts were octagonal because the octagon symbolizes renewal, rebirth, regeneration, and transition, and as Jay Kappraff has pointed out, “the star octagon, an ecclesiastical emblem, signifies resurrection. In medieval number symbolism, eight signified cosmic equilibrium and immortality.”[6] But were these more religious and metaphysical issues of interest to Leonardo? This symbolism may be tied into the centrally designed church that utilized octagonal symmetry, but it may be difficult to say with complete confidence that Leonardo used the octagon for these reasons. Yet, it is certainly possible.
Turning our attention to the mathematical qualities of the octagon, we find that Hermann Weyl, the great twentieth century Princeton mathematician, made an important observation about the types of centrally designed churches that Leonardo was designing. In his book, Symmetry[7], Weyl writes,
In architecture, the symmetry of 4 prevails… Central buildings with a symmetry of 6
are much less frequent… The first pure central building after antiquity, S. Maria degli
Angeli in Florence, is an octagon. Pentagons are very rare.
The octagon, by extension of the square, is then a structure that provided the Renaissance builder with one of the best geometric solutions for centrally designed ecclesiastical architecture where harmony could be maintained between the center and the immediate surrounding plan elements. Weyl goes on to say that,
Leonardo da Vinci engaged in systematically determining the possible symmetries of a central building and how to attach chapels and niches without destroying the symmetry of the nucleus. In abstract modern terminology, his result is essentially our table of the possible finite groups of rotations (proper and improper) in two dimensions. (fig. 3)
Fig. 3. Homogenous articulation of an octagonal plan, after Leonardo, Cod. Atl., fol. 37 r-a; graphite on paper
In other words, the octagon gives the architect a broad range of spacial developments around a central design. And as we can see in many of Leonardo’s octagonal drawings, he experimented widely with the many possibilities afforded by this shape. Within the limits of the two possible symmetry operations, Leonardo was able to come up with a great many variations, each with its own characteristics that made it unique within the general group.
Leonardo himself had suggested at one point that his geometric constructions were to be seen as “geometrical recreations”, but this could have been just a rare moment of lighthearted sarcasm on his part, for Weyl goes on to discuss, “Leonardo’s Theorem.” Leonardo devised a theorem on symmetry in which it is stated that,
“…If improper rotations are taken into consideration, we have the two following possibilities for finite groups of rotations around a center O in plane geometry, which correspond to the two possibilities we encountered for ornamental symmetry on a line: 1.) the group consisting of the repetitions of a single proper rotation by an aliquot part of α = 360dgr/n of 360dgr, and, 2.) the group of these rotations combined with the reflections in n axes forming angles of ½ α. The first group is called the cyclic group, Cn, and the second group the dihedral group, Dn. Thus these are the only possible central symmetries in two-dimensions:
(1) C1, C2, C3, … ;
(2) D1, D2, D3, …
…(Where C1 has no symmetry)…[9]
Professor Steven Wassell clarified this for me in a recent conversation. He said, “Cyclic symmetry groups are readily associated with rotational symmetries, whereas dihedral groups contain reflections, or roughly speaking, bilateral symmetries, as well.” The octagon gives the architect or designer a wide range of spacial developments around a central design because it contains both of these qualities of symmetry. The octagon is a very flexible polygon because of both its own internal relationships and also when it joined with the square, the circle, and various rectangles in the plane.
We also cannot overlook the fact that the octagon provides its user with a perfect vehicle for support, especially regarding the issue of “centering” from an architectural and engineering standpoint. Specifically, I am speaking of the octagon as a bracing system for load bearing problems. An important aspect of Brunelleschi’s dome was that it spanned an immense distance without any central support, that is, a “centering” column. This must have been pointed out to Leonardo, and he certainly realized that the column would have been thrust through the center of an octagonal plane had it been necessary, thereby destroying the greatness of the span. An important quality of the octagon is its strength within its combination of vertical, horizontal and 45 degree diagonal armatures, and clearly, it can compete with the spherical dome as well. This fact is beneficial whether the octagonal plane is horizontal or vertical. (I have included a drawing showing Leonardo’s interest in centering structures (fig. 4).) Often, Leonardo would truncate the lower half of the octagon to allow for the placement of a bridge or walkway. Leonardo also favored a 45 degree buttress in structural problem solving, with an obvious exception being the plans for supporting the tiburio of the Duomo in Milan.
Fig. 4. Centering drawing, after Leonardo, Cod. Atl., fol. 200 r-a; pen & ink on red prepared paper
So then, evidence indicates that Leonardo worked with the octagon because it was both structurally sound and because of the broad range of design possibilities it offers. From both utilitarian and aesthetic viewpoints, Leonardo realized that the octagon provided a great service in the area of architectural design, one that he chose to explore and develop throughout his life.
III. Leonardo and the Octagon
“Octonarius primus in numeris cubicis est, aeternae beatiudinus nobis in anima et corpore stabilitatem simul at soliditatem designans.” (“Eight, as the first perfect cube [2³], imprints us in body and soul with the security of eternal beatitude.”) – Gernot of Rechersberg – Medieval German Theologian
When Leonardo went to Florence to live with his father and secure an apprenticeship in Verrocchio’s studio, Santa Maria di Fiore was the talk of Europe. Filippo Brunelleschi had recently completed the dome of Florence’s Duomo, and Leonardo’s early exposure to the octagonal geometry of this structure (he would have been instructed in square root two/”sacred cut” constructions that yield the octagon) and in the Baptistery of San Giovanni are frequently cited as influences that left profound impressions on the young artist (figs. 5, 6).
Fig. 5. √2 and octagon construction, graphite on paper
Fig. 6. “Sacred cut” and √2 geometry in the square, graphite on paper
While working with Verrocchio, Leonardo would have been directly involved in assisting his master in casting the sphere that was to be placed on the cathedral’s lantern, and it isn’t difficult to imagine Leonardo in the dome while this event was taking place, contemplating it all within the eight sails around him. Brunelleschi had worked with octagonal geometry to the point of perfecting it, and in making the octagon a permanent fixture in ecclesiastical building. Filippo had secured the square based geometry of central system churches like the Old Sacristy, and with Santa Maria del Fiore, he established the permanency of the octagon. If we look at the prevailing geometry of this period, Leonardo tended toward shapes that related to and followed this trend. It is reasonable to assume then that the octagonal geometry of one of the great works of architecture and design stayed with Leonardo as he began his own investigations of central-plan architecture and octagonal structures.
At the same time, it should be remembered that Leonardo did not live in a vacuum, and these ideas and uses of octagons did not come fully formed in Leonardo’s fertile imagination. In the magazine, The Nation, (3/7/03), Arthur Danto points out, in his review of the da Vinci show at the Met in New York (2003), “Leonardo da Vinci, Master Draftsman”, that Leonardo was aware of the work of his medieval predecessors, which included artists, philosophers, builders, mathematicians, and religious writers, as well as those involved in the natural sciences and engineering. Danto cites the works of John Buridan, Albert of Saxony, and Nicholas Oresme as being among those to whom da Vinci was indebted. In historical terms then, Leonardo was not that far removed from Medieval intellectual and practical life. It would be fair to think that he was also aware of the religious writings and thinking of St. Augustine and St. Bernard[10], both of whom wrote at some length about the musical octave, relating to the number eight, and the double square, related to two squares, as is the octagon. (As Da Vinci was also a musical enthusiast, the link seems fairly drawn.) Their writings were widely circulated in France and Italy immediately prior to Leonardo’s birth, and were widely circulated within the circles he moved during his own lifetime. The square, too, was held in great regard by philosophers, theologians, and intellectuals. Many thinkers of the twelfth and thirteenth centuries regarded it as the geometrical representation of the Godhead.[11] As we move then into the early sixteenth century, we find that octagonal geometry also occupied a fair share of the pavement designs for the Laurentian Library[12], indicating that it had held its own throughout the development of the Early Renaissance. So we see that square and octagon based geometry made a formidable and pleasing frame for Leonardo’s creative life, and it appears that he welcomed the situation enthusiastically.
As mentioned, Leonardo’s career ran roughly parallel to the development of the theme of the centrally-designed church. The Latin Cross Cathedrals of the Middle Ages had made way for a renewed interest in the ancient Roman temple, a round, or possibly square or polygonal type of space. Specifically, the area of focus in the cross type churches is where the two arms of the cross intersect. Many cross type churches had incorporated the circle, square, and octagon as the shapes most successful in joining these intersecting arms(fig. 7).
Fig. 7. Leonardo: Drawing for a central plan church MS. B, fol. 24 r, pen and ink
This focal point had developed into an area of exploration in and of itself. It can also be seen that these shapes were a natural result and the best solution to the geometric situation created by the intersection of the two arms. The next step in the evolution of sacred spaces in Europe would be the concept of a central plan, and the octagon, for all intents and purposes, was one perfect solution to the exploration.
Of the polygons, perhaps Leonardo recognized that the octagon, the decagon, and the dodecagon are those that appear to face in “all” directions and also have a relationship of four face tangency[13] with the square. If the octagon is selected for a sacred space, and as that sacred space is traditionally oriented to the Cardinal Points, four additional directions (Vitruvius’ Eight
Winds) (11), could also be utilized. This would give the illusion of a welcoming facade gaining one entry and welcome to the space, regardless of one’s point of origin. One of the concepts of the centrally planned church was this welcoming, communal type of plan, and the octagonal structures that fulfilled this goal were beginning to be studied and developed now in Leonardo’s Italy. Michele Furnari, in, Formal Design in Renaissance Architecture[14], states that,
The temple, known only through the many sketches of the Ashburnham Codex, (fig. 8) belongs to a series of studies Leonardo made on the central-plan typology. As Heydenreich points out, this example is but one of many variations on the theme, ranging from simple plans based on a square or circle to richer, more complex ones based on polygonal shapes. These sketches bear precious witness to continuous experimentation with the concept of the central-plan system… As Pedretti reports, “…As a result of his work on the tiburio of the (Milan) cathedral, Leonardo turned his attention to analyze the structure of a sacred building with a central plan, a typology in which the geometric center coincides with the central balance point of mass…” Leonardo began to combine different geometries and multiple models, starting from the core concept of a building composed of a pivotal inner space to which side spaces are added radially and symmetrically… He composed variations of ever increasing complexity: from square to circular plans, from polygonal to lobed ones. The central-plan concept was thus progressively enriched by the addition of new volumes to the central space… The intersection between the basic circular shape of the dome and other figures (squares, octagons, and so on) give rise to a variety of surfaces of cylindrical and spherical derivation.[15]
Fig. 8. Centrally planned church by Leonardo, MS. Ashb. 2037, 5-v; pen, ink, and graphite on paper
Fig. 9. Copy of Leonardo’s sketches of cupolas (Sheet of eight drawings for raising a dome over a square base), MS. B, fol. 10 v, silverpoint on blue prepared paper
Da Vinci was aware of all these aspects of eight-sided geometry, and he was also becoming aware of octagonal architecture in the plans, domes, cupolas(fig. 9), and decorative elements found in the architecture of Northern Italy as well as in those cities with ports of entry from Eastern cultures. Venice was a major example, and we know that Leonardo made a point of traveling there. He would have known that Venetian builders were influenced in part by the arts and architecture of Persia, India, and the Middle East(fig. 10).
Fig. 10. Constructions of the star octagram and the octagon in the circle, graphite
These influences had made their way from the Holy Land and Asia by scholars, crusaders, and merchants to the Lion City. The variety of octagonal designs and their interplay were a key element in the arts, crafts, and architecture of these cultures, and the octagon held a valuable place, not only in Christendom, but also in Middle Eastern and Asian philosophy, spirituality, and symbolism, and there can be no doubt that Leonardo was aware of all these things.
Octagonal architecture could also be found throughout other areas of Italy. Rome had a great share of samples, and Leonardo, while in Rome doing work for the Papacy, visited both Roman ruins and still existant Roman buildings(fig. 11). There, he had the opportunity to draw and study some of them. (Of great interest to me was Dr. Pedretti’s comments on Leonardo’s book of drawings of old Roman architecture and temples.[16] Pedretti indicates that some of these drawings influenced Leonardo’s octagonal designs for the royal palace of Romorantin [15 B, C].)
Fig. 11. Drawing of a Roman temple, Cod. Ambr., fol. 57, graphite on blue paper
As he began to circulate in the highest intellectual and politically powerful circles of his time, da Vinci would have also learned of still other famous octagonal architecture. San Vitale in Ravenna was still high on the list of famous octagons in his homeland, as were other eight-sided masterworks from elsewhere in Europe, like Aachen Cathedral and the Carolingian palace chapel in Germany, and St. Selpulchre in Cambridge, England. Da Vinci also saw some of the octagonal architecture in other cities and towns in Italy during his travels. He was surely interested in the old octagonal “tower of Boethius” and the octagonal plan of S. Maria alla Pertica in Pavia (fig. 12) and he would have either seen a drawing of the structure by Giuliano da Sangallo while both architects were in Milan, or he may have actually discussed it with him.
Fig. 12. Construction of the plan for the Cathedral of Pavia, after Leonardo, MS B fol. 55 r, pen & ink
It was also during this period that Leonardo took up the idea of designing portable pavilions for the Duke of Milan so that guests could be entertained during festivities outside the pressures of the city, or to be placed in the Duke’s private garden within the formidable walls of the castello[17]. Giuliano had also done some work in this area, and both artists had seemed to find that the octagon and the square were the best geometric structures for this purpose. Of interest in this current study is the drawing da Vinci made of a plan and elevation of a pavilion in the garden of the Castello in Milan: MS. B, ff 11 v- 12r(fig. 13). There are no less than three octagons drawn out related to this project, indicating that Leonardo fully intended to have his octagonal studies reach fruition as an architectural reality for the Duke.
Fig. 13. Temporary architecture for festivals, Cod. Atl. Folio 3 v-b
Da Vinci, as far as is known, had no real formal instruction in geometry. He did, however, have a small collection of books on the subject. Among these texts were Nicholas of Cusa’s (1401-1464), De transformationibus geometricus, and Euclid’s, Elements. He probably read some of the writings by the great Greeks: Archimedes, Theon of Smyrna, Apollonius of Perga, and perhaps even Pappas, Ptolemy, and Nicomachus. But texts like these would probably not have been of much help to him in his investigations specifically into the octagon, except where it may have been of some insight into approximations for pi and for possible solutions to the problem of quadrature. (In early approximations for pi, doubling the square – of which the octagon is the first step from square in the series – within a circle was a technique that was also examined as possibly an aid in squaring the circle.) However, books like Leon Battista Alberti’s treatise on architecture, De re aedificatoria[18], and Francesco di Giorgio’s, Trattato do Architettura Civile e Militare, that Leonardo annotated, were far more useful because artists and architects at that time were versed in geometry not as a mathematical endeavor necessarily, but as a tool to be used in design, composition, perspective, engineering, and architecture. It would be from this position that Leonardo would most likely pursue his interests in things geometric.[19] (Leonardo’s keen eye may have also picked up on one of di Giorgio’s pages of geometry and writing, a page of regular polygons with the glaring omission of the octagon while drawing all of the other shapes; curious to say the least!) Those artists who also were gifted in the mechanical sciences might study geometry for other, more technical reasons, but in the context of the many drawings we find in Leonardo’s journals, it is from an artist’s perspective that we can best understand Leonardo’s work with the octagon, for Leonardo was first and foremost an artist. By the time he worked with Pacioli, he had rendered countless octagonal constructions, and almost all of them freehand, another point that lends itself to an artistic appreciation of his geometric studies. Certainly, Fra Luca enlightened his mind with even more things numerical and geometric, but Leonardo learned them, heard them and saw them, foremost, through the heart and soul of an artist.
Regarding Alberti, when Leonardo examined his writings, he would have reviewed the drawings of the various orders of columns, the plan views of the orders being based on octagonal symmetry with alternating 45° radials emanating from the central vertical axis of the shafts. Of greater importance, I believe, would have been Leonardo’s reading of Alberti’s concise but nonetheless thorough explanations for several methods of constructing octagons in Book Seven, Chapter Four of ’The Ten Books’ , and also Alberti’s efforts in determining the various rotational symmetries of the octagon. (However, it should be remembered that it was Leonardo, not Alberti, who actually put a theorem to these symmetrical operations[20].) Alberti’s influences may be read into some of Leonardo’s drawings – the compositions, layouts, alternations, and ordering of the tribunals, chapels and apses would have benefited Leonardo in his own design quests. Among these influences, we find the following quote by Alberti that Leonardo probably read and considered utilizing:
“I would have nothing on the walls or floor of the temple that did not have some quality of Philosophy… I strongly approve of the patterning the pavement with musical and geometric lines and shapes so that the mind may receive stimuli from every side.”
…And what better representation of the musical octave, as mentioned, than the octagon, and what better way of using the octagon and square [21](the other being hexagonal[22]) than by way of grids, patterns, and tessellations [18]? Although Leonardo saw the idea of tessellation as a decorative device, (figs. 14, 15) , he also saw it as a solution to inhabitable space other than religious, from palazzo to temporary pavilion(fig. 16).
Fig. 14. Tessellation after Leonardo, Cod. Atl., fol. 342 v-b, graphite and chalk on gray tinted paper
Fig. 15. Decorative patterns/geometric construction after Leonardo, pen and ink
Fig. 16. Geometric study after Leonardo on multiple palazzo design, Cod. Atl., fol. 349 v-k and v-c, silverpoint and sepia ink on brown prepared paper
Leonardo was long a lover of nature, a stalwart student of its structures and a tireless witness to its workings. With his great skills of observation, he would know early on that octagons, as a rule, and things referenced by the number eight, are somewhat rare in the natural world. Spiders, sponges, and octopuses aside, octagonal structures are mainly the logical result of the geometric relationships that exist and develop between: a.) the square and the circle; b.) the side of a square and its diagonal; and, c.) combinations of these things along with the interplay of the diagonals and the vertical and horizontal midlines of the square(fig. 17).
Fig. 17. Geometric constructions of square, √2 rectangles, and octagons, graphite
These things are geometric, not natural, and so with the few aforementioned items and with the major exception of the octave in music, we could say then that octagonal systems are mainly a human construct, and we find them frequently used in those contexts. In Leonardo’s day, horoscopes [21] were drawn not as they are today, but in squares, with diagonals and squares rotated through 45 degrees, the basic structure of octagonal symmetry. European philosophers emerging out of the Middle Ages discussed the four seasons and the “Four Cross Quarters” marking the eight major points of a year, the four elements plus their four states diagrammed within an octagon, and the eight major winds of Vitruvius; and on the subject of Vitruvius, we need only look at Leonardo’s now famous Vitruvian Man to grasp the idea. Vitruvius stated that the outstretched arms of a man are very nearly equal in length to that figure’s height; that is, he fits into a square. And when his arms and legs are outstretched to the sides, he fits into a circle. If we reduce these two positions to mathematical signs, the human figure assumes the plus sign and the multiplication sign. Translated to the anatomy of a square, these two symbols are then the midlines and the diagonals in the square’s make up . A contemporary of Leonardo’s, Agrippa von Nettesheim (1486-1535), in his work, De occuta philosophia, went so far as to keep the figures separate, and the two signs are even clearer in his drawings, as Agrippa also drew them with the geometry being discussed here. [22, 23]
Fig. 18. Sheet of constructions of irregular octagons, graphite
Fig.19. Drawing for a central plan church, Cod. Ashb. II, fol. 4 r; graphite on paper
It was a natural development for Leonardo to explore this octagonal geometry formed from the square. This is especially true if we are to believe Leonardo’s own opinions about his rudimentary skills with geometric systems, and that is that he saw his geometric drawings as mentioned, as “geometrical recreations.”[23] The square is universally known, and even people who loosely draw freehand will have, at one time or another, also sketched this very same geometric system of diagonals and midlines in the square. But for Leonardo, it was to be much more than what we think of doodling. Even if he halfheartedly believed his comment, the developed thumbnails were far more advanced than simply light sketching. A close examination of Da Vinci’s octagonal constructions shows that he was quite aware of the very different results obtained when working with the regular octagon as contrasted with irregular ones(fig. 18). His variations included eight sided figures using thirds of a square as well as using different rectangles to form crosses, connecting their corners to make the eight sides. As can be seen in the drawing in the Codex Ashburnham II, fol. 4-r (fig. 19), these irregular octagons are deliberate studies as opposed to random explorations, demonstrated convincingly in the beauty of the resulting structures.
Recently, I saw a documentary film on Leonardo’s famous portrait of Ginevra de’ Benci. In it, Professor Martin Kemp was being interviewed regarding his views on Leonardo and the portrait. Professor Kemp was quick to point out the similarities between the appearance of the spirals of Ginevra’s hair and Leonardo’s renderings of flowing water. He stated that Leonardo was always interested in finding connections between things seen in nature, and, in fact, he had a profound desire to unlock the mysteries of nature through the vehicle of visual art. This rarity of octagons in nature… could this then be the link between Leonardo’s explorations of octagonal symmetry and the fact that there are so few examples of the octagon in the natural world? Simply, there is something rather unique about octagons, and Leonardo must have noticed it. So much so in fact, that even after he was exiled from Italy, in his last three years in France, Leonardo was still developing work on octagonal themes for the King of France while developing drawings for a royal palace at Romorantin [27]. The studies are striking in their beauty and variety, and clearly demonstrate Leonardo’s complete control and mastery over the octagon. They also support the fact that Leonardo, even at the end of his career, was still showing his preference for this shape, and the fact that the drawings are not simply reworkings of older studies adds yet further support.
IV. Commentary on the Drawings
Over this year, I have spent a good deal of time copying a number of Leonardo’s centrally planned cathedrals, many of them octagonal in shape. With these, I also drew other octagonal geometry that he had rendered, unsure if it were a dome, a floor tile, an apse, a baptismal font, or a window, as he did not always label the drawings’ utilitarian functions. On rare occasions, Leonardo took the time to ink up the compass nib and scribe a true circle or arc, leaving no doubt as to the geometric intention. More rarely, and quite helpful, were the countable, evenly spaced calibrations that could be followed, almost like graph paper. Sometimes, there were steps in alphabetical or numerical order. In some of the drawings, Leonardo’s rapidity was such a key quality that I had no hope of unraveling the sketch without making assumptions and studies of my own. There were times I gave the construction a more finished look, with the intention of clarification rather than decoration or embellishment. Some sketches had precedents that I could refer to; some did not. Others were rooted in real buildings and objects, like the studies of the tiburio of the Milan Cathedral, or a tessellation found in Venice. There is a formidable array of recognizable, readable, and solvable octagonal geometry, and I have tried to pick the best of them, and, in my estimation, the more intriguing and the more beautiful of them, to present.
After reviewing about one hundred and twenty drawings, I entered about thirty or so of them into a working journal. I wanted to categorize the drawings and then do precise geometric drawings from the sketches in order to see them as formal architectural renderings or geometric constructions. This would be my best way to establish Leonardo’s intentions and goals with his drawings. As a result of Leonardo’s focus on centrally designed plans, that is, with a clearly defined center and symmetry, some of the drawings look like Tibetan and Indian mandalas, others look like contemporary fractal geometry, some appear as tessellations, while yet others appear to be illustrations for mathematical principles. Of course, each drawing has at least a couple of these qualities present.
Because of the levels of complexity in the drawings, grouping them became necessary for me, and I came up with the following general categories:
1. Mandalas and Tondos (fig. 20a)
2. Fractals (fig. 20b)
3. Satellites: half-circles, circles, squares, octagons, etc. (fig. 20c)
4. Alternations (opposing satellites) (figs. 20d, e)
5. Irregular octagons
6. Tessellations
7. Domes and cupolas
8. Quatrefoils and lunes (fig. 20f)
Fig. 20a. Plan for a many domed church (MS. B., fol. 35r); Venetian red pencil on cream colored paper
Fig. 20b. Project for a centrally planned church (Louvre 2282, reverse); brown ink on blue paper
Fig. 20c. Plan of a many-domed basilica on a square plan with four apses (MS. B, f. 22 r); graphite, pen/black ink on paper
Fig. 20d. Geometric construction after Leonardo, graphite
Fig. 20e. Centrally planned church. (Louvre 2282, obverse); mixed media on paper
Fig. 20f. Geometric construction after Leonardo (lunes) conte crayon
This is no means a complete listing, but it is a relatively good start from all that I saw. Some drawings stand alone, without a group to fit into. Others were done quickly, without any real forethought, and as such, I thought it best not to include them here. Sometimes his drawings were incomplete (a general criticism of Leonardo found even in his octagons!), and there were some that lacked any precision or direction. If I had a reasonable hunch about the intentions in the drawings, even though it was clear that everything was not precisely drawn out, I would make the adjustments in my constructions. To my benefit, one of the results of having to work this way was that I had to draw out a variety of possibilities and variations, thereby deepening my knowledge of the way Leonardo thought geometrically. There were times, too, that it was difficult to determine whether Leonardo had drawn an octagon or a circle for a baptismal area, or a square or a rectangle for a tribune. Because of the interconnectedness of shapes, sometimes he would draw both circles and octagons as parts of the same plan, and it was easy to understand that he was trying out different ideas with different shapes within one general framework. Because a straightedge was not always employed, parts of the plans did not align, and it was sometimes difficult to surmise where a wall was intended to end, a doorway to begin, what was connected to what, and so on. In a couple of the drawings, I kept some of the preliminary linework in the grid and construction lines visible so that the geometric system being presented will be more clear to the viewer. As a result, there are certain passages that had to be interpreted. In those cases, all attempts were made to have the finished drawing look as much as possible like the sketch on which it is based. It was not difficult to understand what Leonardo intended, even though his sketches were occasionally “incorrect”, geometrically. Although perhaps appearing complex, Leonardo’s drawings were not wrought with a great deal of detail nor an abundance of line work, and as such, were relatively easy to follow.
I have not found a good collection of finished geometric drawings done from Leonardo’s architectural studies in any one location, and I believe that it is important to begin one, and this is one of my reasons for cataloging the drawings presented in this folio, and labeling them Books I, II, and so on. I believe that my experiences drawing geometrically can help others to gain some insights not only into Leonardo’s creative oeuvre, but also into an understanding of octagonal symmetry. This understanding may provide some assistance in following Leonardo’s thinking as he went through his work in this area of study, and it may suggest that more study be done specifically on the geometric aspects of Leonardo’s art.
Before concluding, and on a lighter note, it is interesting to me that as there is a direct link between the octagon and the Maltese Cross, and that such a link might provide fuel for those who are interested in conspiracy theories regarding certain secret societies. Much has been made over the past several years about Leonardo’s links to certain mystical fraternities, the Knights of Malta chief among them. I have included a drawing of this cross as used in the official Knights of Malta website (fig. 21).
Fig. 21. Geometric constructions of a Maltese Cross, graphite
The second of the two is a variation. Indeed, the cross displayed on the site is constructed from the octagon, precisely. I say precisely because there are a variety of ways to construct what we call Maltese crosses, yet the official cross on the site is based on the regular octagon, and not on the more popular versions using the equilateral triangle/square root three geometry or the golden section.
V. Conclusions
Leonardo Da Vinci was a singular man of immense intelligence and ability. Even when selecting some detail of the master’s work to examine, his enormity is still felt. It was this aspect of him that stayed with me as I looked over all the geometric sketches and constructions of octagons that he produced. My initial question regarding why he did so much with octagons above and beyond any other geometric shapes can perhaps be answered by historical precedents, the then current trends, and the evidence of his sensitivities with symmetry operations. But I believe it was more than that. Leonardo’s nature, bounding with curiosity and inventiveness, also played a major role. He recognized in the octagon its unique and particular structure in unifying circle and square, square to square, and square to rectangle. He recognized its relationships with other geometric shapes and systems, its potential for a wide variety of design possibilities, and the specific solutions it offered to spacial problems. Brunelleschi’s Dome demonstrated for him that among the geometric shapes at his disposal there were few shapes grander and stronger than the octagon. If Filippo’s Dome had not convinced him, then Bramante’s opinio[25] regarding the work Leonardo was doing on the Milan Cathedral solidified the octagon’s strength and architectural qualities for him[26]. With all this, the octagon also satisfied the soul on symbolic and spiritual levels. This combination of factors gave Leonardo all he needed. There was really little else to do than to explore the myriad of possibilities present in octagonal geometry, and that is exactly what he did.
What we see in the journals is Leonardo at his best, the restless, curious, creative genius, making clay of the octagon, shaping it, bending it, rearranging it, and building with it. His approach to embroidery and knots had been similar, only with them he had recognized another quality of symmetry – that of tessellation – and chose the hexagon and the equilateral triangle for his system. In a similar way, he chose the octagon for his architecture. There was never any doubt in his mind that the octagon was not the best shape for him to work with architecturally. We do not see explorations with a variety of shapes. It was a prime example of Leonardo’s “variations on a theme,” and in this case, that theme was the octagon.
Speaking as an artist and geometer, it seems logical to me that Leonardo would select the octagon as that shape to most closely examine and develop, especially in light of his goals and philosophies regarding architecture. The octagon relates directly to his idea of the ideal central plan temple/church because of its relationship to the circle and the square, and how well the three work together. Additionally, Bramante and Leonardo had both spoken of the supremacy of the square, and the octagon is directly related to it, not only by containing two squares in its structure, but also because it is related to ad quadratum geometry and the ninety degree angle. Circular and square spaces had been built with regularity in antiquity, and the octagon had begun its presence only a short time before Brunelleschi and Leonardo took up the cause. Leonardo apparently did not want to get too involved with ad triangulum geometry, architecture that used equilateral triangles and hexagons. Had he, we would see more hexagonal temple designs in his drawings. By its nature, ad triangulum work presents a different set of building procedures and problems, procedures that Leonardo perhaps felt were too bothersome to deal with. The dodecagon, related to both the square and the triangle, was perhaps a bit too overwhelming and too similar to the circle. (In other words, why work with the twelve sided figure instead of the circle?) The twelve sided figure seems not to have been considered very much, perhaps because it is difficult to draw and handle, and too complex in its internal workings. It is possible that Leonardo saw the dodecagon as too grandiose and impractical, a shape without much architectural history. The pentagon and the decagon were problematic in that they are in conflict with the square and the ninety degree angle, and would have created numerous problems not only in rapid drawing and study, but also in designing and building. Simply stated, the octagon is relatively easy to draw, and once this aspect of it is mastered, the octagon provides perhaps the greatest wealth of geometric possibilities and designs of all the regular polygons after the square. The octagon had a proven historical record, and it is also possible that Leonardo was simply carrying on the tradition of working with it, although with the great number of drawings he did with it, and the great number of variations he produced, I do think he realized that he was taking the shape farther than his predecessors.
Even if we are to believe that Leonardo was being totally sincere about his lack of ability in geometry, the question still needs to be asked: Which aspect of geometry was he speaking about? The proofs, theorems, postulates and mathematical aspects (remembering that mathematics was not a forte of his either) of the subject, or those qualities that attract artists, designers, and architects? My guess is the latter. There is something about geometry and artistic creativity that go hand-in-hand. I believe that it was this aspect of geometry that attracted Leonardo more than any other. Having said that, I believe that the symmetry operations to be found in geometry ran a very close second in Leonardo’s mind, and the symmetry operations of the octagon suited him best of all the simple regular polygons. This can be witnessed in a review of the voluminous geometrical drawings he did. His investigation into the octagon, as found in the drawing in the Windsor Library, Windsor RL, 12700 (fig. 22) is analytical and scientific, to be sure, yet not really mathematical. (This same thing is true when we look at any of his sheets of geometrical drawings: there is little of mathematical notation and a great deal of drawing and quick sketching.) His analysis is one born of a natural curiosity, and one that lends itself to the use and development of geometric constructions in one’s artistic and architectural work.
Fig. 22. Geometric construction after Leonardo, pen & ink
Fig. 23. Geometric construction of an octagon from ad triangulum and ad quadratum, by the author, graphite
Architecture stands between the twin pillars of art and number, and the two do not necessarily have to be separated to be viewed. They are linked by geometry, the visual presentation of numbers (fig. 23). However, when we look at Leonardo’s codices, it would be safe to say that art, rather than mathematics, dominates his work. Whether Leonardo trusted the structural strength and integrity of the octagon as an architectural device because others’ efforts had already demonstrated these qualities to him, or if he had known by mathematical study and discourse that the reliability of the octagon was above question, we may not ever know with certainty. We do not find a great deal in the way of this type of mathematical inquiry in his journals: that is, the use of Euclidean polygons as architectural elements based on their geometrically mathematical qualities. We do find a great many octagonal sketches and studies and sheets of other geometric systems and constructions, but without Euclidean notations and proofs. What we see is that Leonardo was comfortable enough with his knowledge of geometry in general and the symmetry of the octagon in particular to draw it freehand a great majority of the time. It was this overriding interest in the drawings that demonstrates for us his desire to see how the geometry worked. As mentioned, if we are to believe Leonardo’s self-evaluation regarding things geometric, he arrived at his theorem on symmetry by drawing out the octagon in an almost endless variety of ways, and then observed those relationships. It was not by calculation but by Leonardo’s observational skills and artistic ability that brought him expertise in working with geometric elements. This study may only reinforce the obvious suggestion that architects, artists, scientists, and mathematicians would do well to learn from Leonardo. He showed us that geometry provides us with a tool for artistic creativity [32] and a sourcebook for design as well as securing for us the structural and mathematical aspects of masterful architectural forms. Still, there can be times when we would benefit from a study of a work of design from Leonardo’s journals, not by studying the mathematics it contains, but rather by the geometry that was used. If that geometry, based on the user’s expertise (or lack thereof) in mathematics, does not meet exact mathematical standards, it should reflect no less on its user’s knowledge and abilities. There is a difference between geometry as a branch of mathematics and geometry as it is drawn and used in the physical world, especially in the practice of the arts. I believe Leonardo was aware of this. It is interesting to look at his approach to design in light of the fact that he did not believe himself gifted in the discipline of geometric constructions, yet he recognized that by its nature, geometry works wonders with a creative and artistic mind, and so he used it routinely in that spirit. In our efforts to be so specialized today, it seems at times that we may be creating a chasm between the philosophies and practices of art and science. It could be that geometry was more or less abandoned in favor of more advanced realms of thinking and practice before yielding all its treasures to the artist and the scientist, and it could be beneficial to take Leonardo’s exploratory approach, and with his foundation of working with basic geometry. Perhaps Leonardo’s drawings demonstrate that geometry, especially the simple, regular polygons and systems favored by ancient and classical architects, can provide all of us with a bridge to span that chasm.
Author’s note: In Part 2 of this study, I will go more into the geometric principles of the octagon, how it is constructed, and the various relationships that are generated from the basic armature of the structure.